Just give them a calculator
If you don't, students' attention will never be where we need it to be
Struggling with fractions is a common challenge, but Eedi helps address common misconceptions head-on. Use our targeted practice quizzes, automated feedback, and retrieval practice tools to support your students’ understanding - explore The Eedi Collection by logging in or signing up for free by clicking here. Search 'fractions' to find our FREE fractions quizzes.
Once upon a time
Last week, I observed a Year 10 bottom set being taught how to add and subtract fractions with different denominators.
By the end of the lesson, around half the students could successfully add 2/5 and 3/4, whereas the other students would forget the procedure's vital steps and thus get the question wrong. Chatting to the teacher afterwards, she said, with sadness, that the half who can do it now will also have forgotten it by next week.
Looking at the Primary Maths Programmes of Study, students first encounter adding and subtracting fractions where one denominator is a multiple of the other in Year 5, and then adding any two fractions (including mixed numbers) in Year 6. So, this group of students has been taught the same thing for five years and still cannot do it.
This is a problem I see - and have experienced with my students - time and time again. It is a problem not limited to adding and subtracting fractions. Students are taught key procedures repeatedly, yet, to everyone’s frustration - because, believe me, the students feel this as acutely as we do - it does not stick.
Why does this happen?
Many teachers put this down to a memory issue: these students - the ones in the bottom sets - simply cannot retain the steps of these procedures.
But I am not so sure.
I had the privilege of sitting next to one of the Year 10 students throughout the lesson. She was kind enough to talk me through her struggles and let me take photos of her mini-whiteboard.
Her first area of difficulty was finding the lowest common multiple. Here is her board when doing so for 2/5 + 1/4:
This took her just under two minutes to complete. She was fine with the 5-times table, but as you can tell from her rubbing out, the 4s stumped her. I watched her count in steps of 4 on her fingers. Sometimes, she started counting on the previous number instead of counting jumps, so she ended up one number short.
Not only did this take time, it also sapped her attention. Hence, when she finished her list, she couldn't recall the next step: finding the lowest number that appears in both lists.
Things continued in this vein in the next step of the procedure, where students had to multiply the numerators to create a pair of equivalent fractions:
Here, she has made two mistakes on the right-hand side: what to multiply by and what the resulting numerator is. She explained that she wasn’t very good at timesing.
When we corrected this, I had to remind her what to do for the next step of the procedure - add the numerators of the two fractions - even though the teacher had gone through a similar example a few minutes ago.
I hypothesise that the issue is not some inability of a certain type of student to retain information. It is because students’ attention has been diverted away from what they need to be thinking about - the steps of the procedures - because so much attention needs to be expended on the arithmetic. As we know from Dan Willingham’s work, memory is the residue of thought. So, if students do not think hard about the procedure to add and subtract fractions, then it is little wonder that they cannot retain it.
So, what is the solution?
I think there are two things teachers can do.
First, keep the numbers as simple as possible. 2s, 5s and 10s are usually safe bets. This is a good strategy for all classes and for all topics, but especially for those students who struggle with arithmetic and those procedures with multiple steps. Only increase the complexity of the numbers to the levels students would typically meet in an exam when the steps of the procedure have been automated and so require less attention.
Second, give students a calculator and let them use it whenever they want. Interestingly, I suggested this to the member of SLT who was watching the lesson with me, and her response was: But adding fractions is going to be on the non-calculator exam paper, so students need to practice doing it without a calculator.
This is the wrong way to think about it. Let’s take it to the extreme and suppose that, given enough attention to arithmetic, students arrive at the exam able to do things like 4-times table and basic multiplication, giving it little thought. That knowledge is useless because they have not given sufficient attention to the procedures where they need to apply these skills, so they can’t answer any of these questions.
Instead, if we allow students to use their calculator whenever they like - and openly encourage it - students offload the burden of doing the arithmetic. They can dedicate more of their attention to the steps of the procedures - both the order and why they are doing them. Thus, when they take the exam, their arithmetic may still not be where it needs to be, but because they can recall the steps of the procedure, they have a better chance of success.
There is a second reason to encourage regular calculator use. Students who most need a calculator are often the least proficient in using one. Anyone who has marked calculator papers at GCSE will see low-achieving students use written methods for multiplications that they could just put in their calculator. Repeated practice is the only way to build this much-needed awareness and proficiency.
Of course, we need to be careful. To take the adding fractions example, we want students to use their calculator to support them in doing the individual steps of the procedure - listing multiples, multiplying numbers to find equivalent fractions - and not just typing 2/5 + 1/4 and getting the answer immediately. But we can ensure this by checking each step of the process on mini-whiteboards and insisting on full working out.
One final point. A calculator should play a key role in all maths lessons for all students, regardless of the topic. Let’s imagine we have a top set doing the adding and subtracting fractions lesson. They will not need to use a calculator to do each step of the procedure because multiplication facts have been automated to such an extent that they require little attention. However, these students should be encouraged to check their answers using their calculators - so, type in 2/5 + 3/4 and see if the calculator agrees with them. This serves two purposes: it builds calculator proficiency and makes checking answers more efficient than students having to wait until the end of the lesson.
In summary…
When students learn a new idea, we want as much of their attention to be on that idea as possible. If a lack of arithmetic skills will divert their attention away from thinking hard about the idea, offload that attention by giving them a calculator.
What do you agree with, and what have I missed?
Let me know in the comments below!
🏃🏻♂️ Before you go, have you…🏃🏻♂️
… checked out our incredible, brand-new, free resources from Eedi?
… read my latest Tips for Teachers newsletter about engaging silent students?
… listened to my most recent podcast about the Do Now?
… read my write-ups of everything I have learned from watching 1000s of lessons?
… considered purchasing one of my new 90-minute online CPD courses?
Thanks so much for reading and have a great week!
Craig

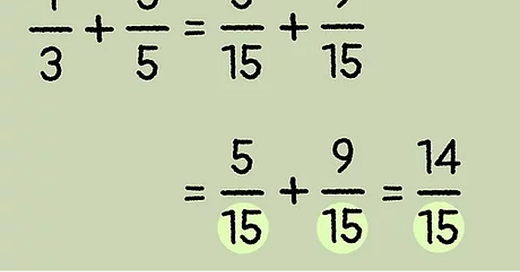




I thought this was very interesting, and will try to make more use of it. My school does the MYP, which is exclusively calculator, but embedded in the computer-based response programme, so has different functionality.
I cam to the comments to say that if teachers were concerned about students "cheating" by using a scientific calculator, there are many occasions where a basic calculator could be offered for the arithmetic, without this being able to skip steps in the larger process.
Completely sensible. Before being a Maths teacher I had worked in coal mines, building and installing machinery. It was thought macho to crawl down a coal face 3ft high without kneepads. In the US where productivity was far higher no such macho nonsense prevailed and people had decent kneepads. I always felt that letting students in study and learning use calculators allowed them to use their own initiative and feel ownership. Where in any work environment now would you trust someone who said he would ignore his calculat(or/ing machine) and do the sum in his head? I wouldn’t even trust myself if I had a calculator to hand. If we are to teach students to go into a joined up world and work in teams, non-calculator is simply non-sense!